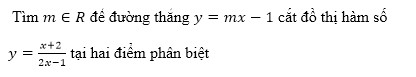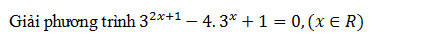Số nghiệm thực của phương trình 4^x - 1 + 2^x + 3 - 4 = 0 là
Câu hỏi
Nhận biếtSố nghiệm thực của phương trình \({4^{x - 1}} + {2^{x + 3}} - 4 = 0\) là
Đáp án đúng: A
Lời giải của Tự Học 365
Giải chi tiết:
Ta có:
\(\begin{array}{l}\;\;\;{4^{x - 1}} + {2^{x + 3}} - 4 = 0 \Leftrightarrow \frac{1}{4}{.2^{2x}} + {8.2^x} - 4 = 0\\ \Leftrightarrow \left[ \begin{array}{l}{2^x} = - 16 + 4\sqrt {17} \;\;\left( {tm} \right)\\{2^x} = - 16 - 4\sqrt {17} \;\;\left( {ktm} \right)\end{array} \right. \Leftrightarrow x = {\log _2}\left( {4\sqrt {17} - 16} \right).\end{array}\)
Vậy phương trình đã cho có 1 nghiệm.
Chọn A.
Luyện tập
Câu hỏi liên quan
-
Trong không gian với hệ trục Oxyz, cho mặt phẳng (P): 2x + y + 2z + 4 = 0, đường thẳng d:
= 
= 
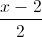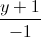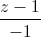và đường thẳng ∆ là giao tuyến của hai mặt phẳng x = 1, y + z - 4 = 0. Viết phương trình mặt cầu có tâm thuộc d, đồng thời tiếp xúc với ∆ và (P) biết rằng tâm của mặt cầu có tọa độ nguyên.
là số thực và z2 =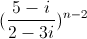 
là số ảo.